

By Courtney Taylor. Statistics Expert
Courtney K. Taylor, Ph.D. is an associate professor of mathematics at Anderson University in Anderson, Indiana. He teaches a wide variety of courses throughout mathematics, including those involving statistics.
Updated November 25, 2015.
Hypothesis testing involves the careful construction of two statements: the null hypothesis and the alternative hypothesis. These hypotheses can look very similar when written down, but actually occupy positions in our hypothesis test that are not on the same footing. How do we know which hypothesis is the null and which one is alternative? There are a few ways to tell the difference.
The Null Hypothesis
The null hypothesis reflects that there will be no observed effect for our experiment.
Continue Reading Below
In a mathematical formulation of the null hypothesis there will typically be an equal sign. This hypothesis is denoted by H0 .
The null hypothesis is what we attempt to find evidence against in our hypothesis test. We hope to obtain a small enough p-value that it is lower than our level of significance alpha and we are justified in rejecting the null hypothesis. If our p-value is greater than alpha, then we fail to reject the null hypothesis.
If the null hypothesis is not rejected, then we must be careful to say what this means.
The thinking on this is similar to a legal verdict. Just because a person has been declared “not guilty”, it does not mean that he is innocent. In the same way, just because a null hypothesis is not rejected does not mean that the statement is true.

For example, we may want to investigate the claim that despite what convention has told us, the mean adult body temperature is not the accepted value of 98.6 degrees Fahrenheit.

The null hypothesis for an experiment to investigate this is “The mean adult body temperature is 98.6 degrees Fahrenheit.” If we fail to reject the null hypothesis, then our working hypothesis remains that the average adult has temperature of 98.6 degrees.
Continue Reading Below
If we are studying a new treatment, the null hypothesis is that our treatment will not change our subjects in any meaningful way.
The Alternative Hypothesis
The alternative or experimental hypothesis reflects that there will be an observed effect for our experiment. In a mathematical formulation of the alternative hypothesis there will typically be an inequality, or not equal to symbol. This hypothesis is denoted by either Ha or by H1 .
The alternative hypothesis is what we are attempting to demonstrate in an indirect way by the use of our hypothesis test. If the null hypothesis is rejected, then we accept the alternative hypothesis. If the null hypothesis is not rejected, then we do not accept the alternative hypothesis. Going back to the above example of mean human body temperature, the alternative hypothesis is “The average adult human body temperature is not 98.6 degrees Fahrenheit.”
If we are studying a new treatment, then the alternative hypothesis is that our treatment does in fact change our subjects in a meaningful and measureable way.
Negation
The following set of negations may help when you are forming your null and alternative hypotheses.
Most technical papers rely on just the first formulation, even though you may see some of the others in a statistics textbook.
- Null hypothesis: “x is equal to y .” Alternative hypothesis “x is not equal to y .”
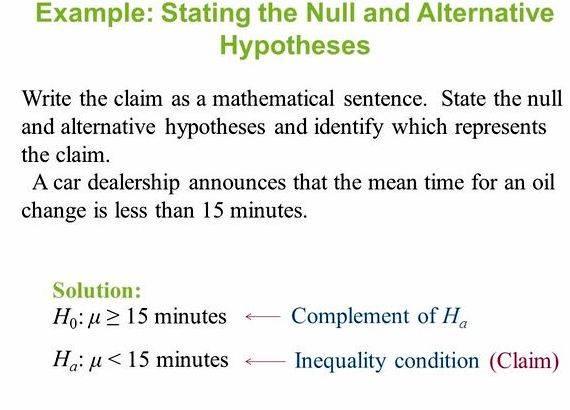
- Null hypothesis: “x is at least y .” Alternative hypothesis “x is less than y .”
- Null hypothesis: “x is at most y .” Alternative hypothesis “x is greater than y .”
Follow This Example of a Hypothesis Test
By Courtney Taylor. Statistics Expert
Courtney K. Taylor, Ph.D. is an associate professor of mathematics at Anderson University in Anderson, Indiana. He teaches a wide variety of courses throughout mathematics, including those involving statistics.
Updated November 25, 2015.
Hypothesis testing involves the careful construction of two statements: the null hypothesis and the alternative hypothesis. These hypotheses can look very similar when written down, but actually occupy positions in our hypothesis test that are not on the same footing. How do we know which hypothesis is the null and which one is alternative? There are a few ways to tell the difference.
The Null Hypothesis
The null hypothesis reflects that there will be no observed effect for our experiment.
Continue Reading Below
In a mathematical formulation of the null hypothesis there will typically be an equal sign. This hypothesis is denoted by H0 .
The null hypothesis is what we attempt to find evidence against in our hypothesis test. We hope to obtain a small enough p-value that it is lower than our level of significance alpha and we are justified in rejecting the null hypothesis. If our p-value is greater than alpha, then we fail to reject the null hypothesis.
If the null hypothesis is not rejected, then we must be careful to say what this means.
The thinking on this is similar to a legal verdict. Just because a person has been declared “not guilty”, it does not mean that he is innocent. In the same way, just because a null hypothesis is not rejected does not mean that the statement is true.
For example, we may want to investigate the claim that despite what convention has told us, the mean adult body temperature is not the accepted value of 98.6 degrees Fahrenheit. The null hypothesis for an experiment to investigate this is “The mean adult body temperature is 98.6 degrees Fahrenheit.” If we fail to reject the null hypothesis, then our working hypothesis remains that the average adult has temperature of 98.6 degrees.
Continue Reading Below
If we are studying a new treatment, the null hypothesis is that our treatment will not change our subjects in any meaningful way.
The Alternative Hypothesis
The alternative or experimental hypothesis reflects that there will be an observed effect for our experiment. In a mathematical formulation of the alternative hypothesis there will typically be an inequality, or not equal to symbol. This hypothesis is denoted by either Ha or by H1 .
The alternative hypothesis is what we are attempting to demonstrate in an indirect way by the use of our hypothesis test. If the null hypothesis is rejected, then we accept the alternative hypothesis. If the null hypothesis is not rejected, then we do not accept the alternative hypothesis. Going back to the above example of mean human body temperature, the alternative hypothesis is “The average adult human body temperature is not 98.6 degrees Fahrenheit.”
If we are studying a new treatment, then the alternative hypothesis is that our treatment does in fact change our subjects in a meaningful and measureable way.
Negation
The following set of negations may help when you are forming your null and alternative hypotheses. Most technical papers rely on just the first formulation, even though you may see some of the others in a statistics textbook.
- Null hypothesis: “x is equal to y .” Alternative hypothesis “x is not equal to y .”
- Null hypothesis: “x is at least y .” Alternative hypothesis “x is less than y .”
- Null hypothesis: “x is at most y .” Alternative hypothesis “x is greater than y .”
Follow This Example of a Hypothesis Test
Previous answers to this question
This is a preview of an assignment submitted on our website by a student. If you need help with this question or any assignment help, click on the order button below and get started. We guarantee authentic, quality, 100% plagiarism free work or your money back.
 Get The Answer
Get The Answer